Dynamic Iteration Schemes
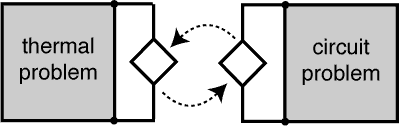
Dynamic iteration via source coupling
Standard time-integration methods solve transient problems all at once. This may become very inefficient or impossible for large systems of equations. Imaging that such large systems often stem from a coupled problem formulation, where different physical phenomena interact and need to be coupled in order to produce a precise mathematical model.
E.g. highly integrated electric circuits (as in memory chips or CPUs) produce heat, which effects in turn their behavior as electrical system; thus one needs to couple electric and thermal subproblem descriptions. On the one hand, this creates multiple time scales due to different physical phenomena, which demands an efficient treatment, see multirate. On the other hand, in a professional environment one usually has dedicated solvers for the subproblems, which need to be used, and an overall problem formulation is not feasible for any of the involved tools.
For those partitioned problems a dynamic iteration method becomes beneficial or even the sole way-out: it keeps the subproblems separate, solves subproblems sequentially (or in parallel) and iterates until convergence (fixed-point interation). Thus the subproblem's structure can be exploited in the respective integration.
To guarantee or to speed up convergence the time interval of interest is split into a series of windows. Then the time-integration of the windows is applied sequentially and in each window the subproblems are solved iteratively by your favoured method.
Group members working on that field
- Andreas Bartel
- Michael Günther
Former and ongoing Projects
Cooperation
- Herbert De Gersem, Katholieke Universiteit Leuven
Publications
- 1986
177.
Beardsworth, R.; Bunker, Philip R.; Jensen, Per; Kraemer, Wolfgang P.
Rotation-vibration energy levels of H\(_{2}\)O and C\(_{3}\) calculated using the nonrigid bender Hamiltonian
Journal of Molecular Spectroscopy, 118 (1) :50-63
1986176.
Beardsworth, R.; Bunker, Philip R.; Jensen, Per; Kraemer, Wolfgang P.
Rotation-vibration energy levels of H\(_{2}\)O and C\(_{3}\) calculated using the nonrigid bender Hamiltonian
Journal of Molecular Spectroscopy, 118 (1) :50-63
1986175.
Tausch, Michael W.
Silberfreie Photographie und Photochromie
Praxis der Naturwissenschaften (Chemie), 35 :19
1986174.
Maten, E. J. W.
Splitting methods for fourth order parabolic partial differential equations
Computing, 37 (4) :335--350
Dezember 1986
Herausgeber: Springer Science and Business Media {LLC}173.
Fink, Ewald H.; Kruse, H.; Ramsay, D. A.
The high-resolution emission spectrum of S\(_{2}\) in the near infrared: The b\(^{1}\)\(\Sigma\)\(_{g}\)\(^{+}\) - X\(^{3}\)\(\Sigma\)\(_{g}\)\(^{-}\) system
Journal of Molecular Spectroscopy, 119 (2) :377-387
1986172.
Fink, Ewald H.; Kruse, H.; Ramsay, D. A.
The high-resolution emission spectrum of S2 in the near infrared: The b1Σg+ - X3Σg- system
Journal of Molecular Spectroscopy, 119 (2) :377-387
1986171.
Jensen, Per; Johns, John W. C.
The infrared spectrum of carbon suboxide in the \(\nu\)\(_{6}\) fundamental region: Experimental observation and semirigid bender analysis
Journal of Molecular Spectroscopy, 118 (1) :248-266
1986170.
Jensen, Per; Johns, John W. C.
The infrared spectrum of carbon suboxide in the \(\nu\)\(_{6}\) fundamental region: Experimental observation and semirigid bender analysis
Journal of Molecular Spectroscopy, 118 (1) :248-266
1986169.
Jensen, Per; Bunker, Philip R.
The nonrigid bender Hamiltonian using an alternative perturbation technique
Journal of Molecular Spectroscopy, 118 (1) :18-39
1986168.
Bielefeld, M.; Wildt, J{ü}rgen; Fink, Ewald H.
Rate constants of the near-resonant E-E energy exchange processes SeS(b0\(^{+}\)) + O\(_{2}\)(X\(^{3}\)\(\Sigma\)\(_{g}\)\(^{-}\)) ↔ SeS(X\(_{1}\)0\(^{+}\)) + O\(_{2}\)(a\(^{1}\)\(\Delta\)\(_{g}\))
Chemical Physics Letters, 126 (5) :421-426
1986167.
Jensen, Per; Bunker, Philip R.
The nonrigid bender Hamiltonian using an alternative perturbation technique
Journal of Molecular Spectroscopy, 118 (1) :18-39
1986166.
Jensen, Per; Bunker, Philip R.
The nonrigid bender Hamiltonian using an alternative perturbation technique
Journal of Molecular Spectroscopy, 118 (1) :18-39
1986165.
Bunker, Philip R.; Jensen, Per; Kraemer, Wolfgang P.; Beardsworth, R.
The potential surface of X\verb=~=\(^{3}\)B\(_{1}\) methylene (CH\(_{2}\)) and the singlet-triplet splitting
The Journal of Chemical Physics, 85 (7) :3724-3731
1986164.
Bunker, Philip R.; Jensen, Per; Kraemer, Wolfgang P.; Beardsworth, R.
The potential surface of X\verb=~=\(^{3}\)B\(_{1}\) methylene (CH\(_{2}\)) and the singlet-triplet splitting
The Journal of Chemical Physics, 85 (7) :3724-3731
1986163.
Bunker, Philip R.; Jensen, Per; Kraemer, Wolfgang P.; Beardsworth, R.
The potential surface of X~3B1 methylene (CH2) and the singlet-triplet splitting
The Journal of Chemical Physics, 85 (7) :3724-3731
1986162.
Vojt{í}k, Jan; Spirko, Vladim{í}r; Jensen, Per
Vibrational energies of H\(_{3}\)\(^{+}\) and Li\(_{3}\)\(^{+}\) based on the diatomics-in-molecules potentials
Collection of Czechoslovak Chemical Communications, 51 (10) :2057-2062
1986
Herausgeber: Institute of Organic Chemistry and Biochemistry AS CR, v.v.i.161.
Vojt{í}k, Jan; Spirko, Vladim{í}r; Jensen, Per
Vibrational energies of H\(_{3}\)\(^{+}\) and Li\(_{3}\)\(^{+}\) based on the diatomics-in-molecules potentials
Collection of Czechoslovak Chemical Communications, 51 (10) :2057-2062
1986
Herausgeber: Institute of Organic Chemistry and Biochemistry AS CR, v.v.i.160.
Vojtík, Jan; Spirko, Vladimír; Jensen, Per
Vibrational energies of H3+ and Li3+ based on the diatomics-in-molecules potentials
Collection of Czechoslovak Chemical Communications, 51 (10) :2057-2062
1986
Herausgeber: Institute of Organic Chemistry and Biochemistry AS CR, v.v.i.159.
Bielefeld, M.; Wildt, Jürgen; Fink, Ewald H.
Rate constants of the near-resonant E-E energy exchange processes SeS(b0+) + O2(X3Σg-) ↔ SeS(X10+) + O2(a1Δg)
Chemical Physics Letters, 126 (5) :421-426
1986158.
Bielefeld, M.; Wildt, J{ü}rgen; Fink, Ewald H.
Rate constants of the near-resonant E-E energy exchange processes SeS(b0\(^{+}\)) + O\(_{2}\)(X\(^{3}\)\(\Sigma\)\(_{g}\)\(^{-}\)) ↔ SeS(X\(_{1}\)0\(^{+}\)) + O\(_{2}\)(a\(^{1}\)\(\Delta\)\(_{g}\))
Chemical Physics Letters, 126 (5) :421-426
1986157.
Jensen, Per; Winnewisser, Manfred
Prediction of higher inversion energy levels for isocyanamide H2NNC
Collection of Czechoslovak Chemical Communications, 51 (7) :1373-1381
1986
Herausgeber: Institute of Organic Chemistry and Biochemistry AS CR, v.v.i.156.
Beardsworth, R.; Bunker, Philip R.; Jensen, Per; Kraemer, Wolfgang P.
Ab initio rotation-vibration energies of HOC\(^{+}\) calculated using the nonrigid bender Hamiltonian
Journal of Molecular Spectroscopy, 118 (1) :40-49
1986155.
Maten, E. Jan W.; Sleijpen, Gerard L. G.
A convergence analysis of Hopscotch methods for fourth order parabolic equations
Numerische Mathematik, 49 (2-3) :275--290
März 1986
Herausgeber: Springer Science and Business Media {LLC}154.
Jensen, Per; Spirko, Vladim{í}r
A new Morse-oscillator based Hamiltonian for H\(_{3}\)\(^{+}\): Calculation of line strengths
Journal of Molecular Spectroscopy, 118 (1) :208-231
1986153.
Jensen, Per; Spirko, Vladim{í}r
A new Morse-oscillator based Hamiltonian for H\(_{3}\)\(^{+}\): Calculation of line strengths
Journal of Molecular Spectroscopy, 118 (1) :208-231
1986
