Coupled DAE Problems
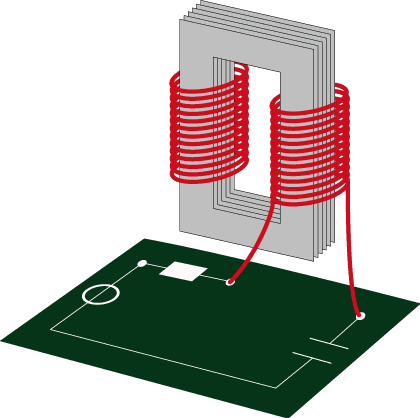
A circuit (DAE model) coupled to a magnetostatic field device (PDE model)
Coupled Problems of differential-algebraic equations (DAEs) arise typically from either multiphysical modeling (e.g. in circuit simulation with heating) or from refined modeling, where crucial parts of the original problem are replaced by a better, but computational more expensive model (e.g. circuits refined by field models). Furthermore splitting methods may turn a monolithic DAE problem into coupled subproblems, e.g. because of different time scales (multirate). In any case the DAEs arise from network approaches or space-discretization of PDAEs (Partial Differential Algebraic Equations).
Often the coupled equations have quite different properties, i.e., symmetries, definiteness or time scales. Thus the coupled system must be analyzed (e.g. the index) and tailored methods have to be developed (e.g. dynamic iteration).
Details
Publications
- 2023
5017.
Schweitzer, Marcel
Sensitivity of matrix function based network communicability measures: Computational methods and a priori bounds
SIAM J. Matrix Anal. Appl., 44 (3) :1321-1348
20235016.
Alameddine, Jean-Marco; others
Simulating radio emission from air showers with CORSIKA 8
PoS, ICRC2023 :425
20235015.
Alameddine, Jean-Marco; others
Simulations of cross media showers with CORSIKA 8
PoS, ICRC2023 :442
20235014.
Giaccari, Ugo Gregorio; others
Simulations of the antenna response for the Auger Radio Detector
PoS, ARENA2022 :042
20235013.
Schweitzer, Marcel
Sketched and truncated polynomial Krylov methods: Evaluation of matrix functions
20235012.
Schweitzer, Marcel
Sketched and truncated polynomial Krylov methods: Matrix Equations
20235011.
Bond, Amelia M. H.; Frey, Markus M.; Kaiser, Jan; Kleffmann, Jörg; Jones, Anna E.; Squires, Freya A.
Snowpack nitrate photolysis drives the summertime atmospheric nitrous acid (HONO) budget in coastal Antarctica
Atmospheric Chemistry and Physics, 23 (9) :5533—5550
May 2023
ISSN: 1680-73245010.
Acu, Ana-Maria; Heilmann, Margareta; Raşa, Ioan
Some results for the inverse of a Bernstein–Schnabl type operator
Analysis and Mathematical Physics, 13 (1)
20235009.
Mui, Jonathan
Spectral properties of locally eventually positive operator semigroups
Semigroup Forum, 106 :460-480
20235008.
Schäfers, Kevin; Bartel, Andreas; Günther, Michael; Hachtel, Christoph
Spline-oriented inter/extrapolation-based multirate schemes of higher order
Applied Mathematics Letters, 136 :108464
2023
Publisher: Pergamon5007.
Schäfers, Kevin; Bartel, Andreas; Günther, Michael; Hachtel, Christoph
Spline-oriented inter/extrapolation-based multirate schemes of higher order
Applied Mathematics Letters, 136 :108464
2023
Publisher: Pergamon5006.
Schäfers, Kevin; Bartel, Andreas; Günther, Michael; Hachtel, Christoph
Spline-oriented inter/extrapolation-based multirate schemes of higher order
Applied Mathematics Letters, 136 :108464
2023
Publisher: Pergamon5005.
Clemens, Markus; Günther, Michael
Stability of Transient Coupled Multi-Model Discrete Electromagnetic Field Formulations Using the Port-Hamiltonian System Framework
2023 International Conference on Electromagnetics in Advanced Applications (ICEAA), Page 1–1
Publisher: IEEE
20235004.
Clemens, Markus; Günther, Michael
Stability of Transient Coupled Multi-Model Discrete Electromagnetic Field Formulations Using the Port-Hamiltonian System Framework
2023 International Conference on Electromagnetics in Advanced Applications (ICEAA), Page 1–1
Publisher: IEEE
20235003.
Muniz, Michelle; Ehrhardt, Matthias; Günther, Michael; Winkler, Renate
Strong stochastic Runge-Kutta-Munthe-Kaas methods for nonlinear Itô SDEs on manifolds
Applied Numerical Mathematics, 193 :196–203
2023
Publisher: North-Holland5002.
Muniz, Michelle; Ehrhardt, Matthias; Günther, Michael; Winkler, Renate
Strong stochastic Runge-Kutta-Munthe-Kaas methods for nonlinear Itô SDEs on manifolds
Applied Numerical Mathematics, 193 :196–203
2023
Publisher: North-Holland5001.
Muniz, Michelle; Ehrhardt, Matthias; Günther, Michael; Winkler, Renate
Strong stochastic Runge-Kutta-Munthe-Kaas methods for nonlinear Itô SDEs on manifolds
Applied Numerical Mathematics, 193 :196–203
2023
Publisher: North-Holland5000.
Muniz, Michelle; Ehrhardt, Matthias; Günther, Michael; Winkler, Renate
Strong stochastic Runge-Kutta–Munthe-Kaas methods for nonlinear Itô SDEs on manifolds
Applied Numerical Mathematics
2023
ISSN: 0168-92744999.
Günther, Michael; Jacob, Birgit; Totzeck, Claudia
Structure-preserving identification of port-Hamiltonian systems - a sensitivity-based approach
20234998.
Günther, Michael; Jacob, Birgit; Totzeck, Claudia
Structure-preserving identification of port-Hamiltonian systems--a sensitivity-based approach
arXiv preprint arXiv:2301.02019
20234997.
Relton, Samuel D.; Schweitzer, Marcel
Structured level-2 condition numbers of matrix functions
20234996.
Studies on the improvement of the matching uncertainty definition in top-quark processes simulated with Powheg+Pythia 8
CERN, Geneva
20234995.
Bolten, Matthias; Donatelli, M.; Ferrari, P.; Furci, I.
Symbol based convergence analysis in block multigrid methods with applications for Stokes problems
Appl. Numer. Math., 193 :109-130
20234994.
Bolten, Matthias; Donatelli, Marco; Ferrari, Paola; Furci, Isabella
Symbol based convergence analysis in multigrid methods for saddle point problems
Linear Algebra Appl., 671 :67--108
20234993.
Bolten, Matthias; Donatelli, Marco; Ferrari, Paola; Furci, Isabella
Symbol based convergence analysis in multigrid methods for saddle point problems
Linear Algebra Appl., 671 :67--108
2023
